Until Copernicus (1473-1543) the prevalent and politically safe
point of view of the universe was geocentric.
Tycho Brahe (1546-1601) recorded positions of Mars and another
planets with instruments developped by himself in the pre-telescope and
pre-pendulus clock era.
Kepler (1571-1630) dechipered the Tycho Brahe observations in terms
of the Kepler's laws. He had to perform a lot
of arithmetical operations to deriver their laws, 400 years before
the computer age. But he dieda poor man...
And Isaac Newton (1642-1727) explained why with the gravitational
law and the two-body problem solution.
Two-body problem: Given at any time the positions and velocities
of two particles of known mass moving under their mutual gravitational
force
calculate their positions and velocities at any other time
Universal gravitation law: Every particle of matter M
in the Universe
attracts every other particle of matter m with a force 
directly
proportional
to the product of their masses and inversely proportional to the square
of
the distance r between them:
being 
m 
kg 
s 
, 
, 
the position
vectors for M and m respectively and 
the relative position
vector. We will consider the body 1 (M) as the Earth
and the body 2 (m) the satellite.
being 
and integrating
we get:
we get
that
the angular momentum per mass unit 
remains constant in the orbit:
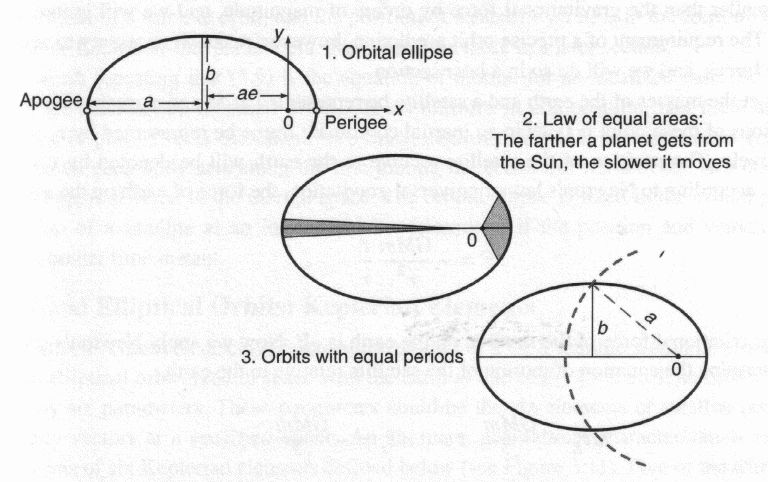
Then the motion of the satellite is performed in the plane
perpendicular to with 
constant, being 
the polar coordinates
of the satellite in this plane (2nd Kepler's law).
and
taking into account that 
:
The double vectorial product of the right term can be developed as:
And a (new) third integral is found:
This is a vector contained in the orbit plane pointing to the
perigee,
which module is the eccentricity. Indeed, multiplying by and
applying the "cyclic" property of the double "scalar-vectorial"
product:
being 
the angle of the position vector referred to the perigee (true
anomaly).
And finally we can get from this expression, the ellipse
equation of parameter 
and eccentricity e:
From these expressions is possible to compute the velocity components in the orbit plane (polar coordinates) and the velocity module:
